It was the Danish astronomer Ole Rømer who in 1767 gave us the data to calculate a meaningful value of the speed of light. Ole had noticed that there were differences in the times when you saw Io, one of Jupiter's moons, start to cross the face of the planet. Sometimes it wasn't on schedule.

Ole then realized that when the Earth was further away, the calculated time would be off since light had further to travel. Ole himself didn't do the calculations, but with Ole's data the Dutch scientist Christiaan Huygens came up with a value of 211,000 kilometers per second. Not bad for the time.

In 1728, the English astronomer, James Bradley gave us a number that came close to the one we use today. He realized that stellar aberration - apparent shifts in the positions of stars - were due to light's finite speed. He calculated a value of 301,000 km/s. That was less that half a percent off.

Then in 1862, Armand Hippolyte Louis Fizeau - his friends called him Louis - decided to measure the speed of light in the laboratory. He crafted a windmill through which he sent a beam of light - kind of like the apparatus in Bonanza - to a mirror 5 miles away. His value was 313,000 kilometers per second. True, that's not as good as what James got. But it was the first measurement of the speed of light that did not rely on astronomical observations.

The best value of the nineteenth century was when Louis Fizeau teamed up with Lèon Foucault who had invented a new device. Lèon's apparatus bounced a beam of light off a rotating mirror to another mirror about twenty miles away. From where the returning beam landed, you could calculate how long the light took to travel the forty miles. Lèon measured light's speed at 299,796 kilometers per second.

Albert used improvements of Lèon's device. Using the skills of master technicians and machinists, Albert had all the stuff made specially for himself. By now Albert had emerged as both a top-notch experimentalist as well as a first rate theorist - sort of an early-day Enrico Fermi. One of his earlier values for the speed of light was 299,338 kilometers per second and he got better and better. The last experiment Albert ran gave a value of 299,774 kilometers per second but he died before he could do the actual calculations.

By 1950, the value had been honed down to 299792.5 kilometers per second with an error of ±1 kilometer per second. Then in 1971 the value had been refined - now using meters - to 299792456.2 ±1.1 meters per second.

Today we know the EXACT speed of light, and we no longer need to measure it. Why? Well, that's because we define the meter as EXACTLY the distance traveled by light in ¹/_299792458 of a second. So the speed of light is EXACTLY 299,792,458 meters per second. That's 299,792.458 kilometers per second or (to use old-fashioned units), 186282.3971 miles per second which is 186,282 miles, 2096 feet, 5 and ^{1,653,528,698}/_{10,000,000,000} inches.

Exactly.

 

Aether Or ... ?

One thing that was puzzling scientists was just how the heck light traveled through space. There was no problem in Isaac Newton's time since they thought light was just particles - that is, everyone preferred Isaac's "corpuscular" theory. But by the nineteenth century, the wave theory of light - advocated by Christiaan - had won out. But that created another problem.

Waves are not actually things. Instead they are a phenomenon that results when a stationary material wiggles back and forth so that it's the oscillation front that moves forward. Many materials can transmit waves. Water works fine for water waves, thank you. And air can produce sound waves.

But what kind of waves are light waves? How could light travel through empty space?

Well, the thinking was that there was no empty space. Instead space was filled with a "luminiferous aether" (as it was spelled, although we'll now opt for the modern spelling). This meant that the Earth, the Sun, and all the planets were traveling through some kind of "ethereal" fluid which itself didn't move.

This gave Albert an idea. He figured that with his optical equipment he could measure the absolute speed of the Earth through the ether.

How so? Well, Albert - and everyone else - knew that if you tossed a baseball from a train, the speed of the ball will be different depending on which direction you threw it. Say as the train moves forward at 60 miles per hour, you toss a 57 mile an hour curveball in the direction the train is moving. The actual speed of the ball will be 60 + 57 = 117 miles per hour.

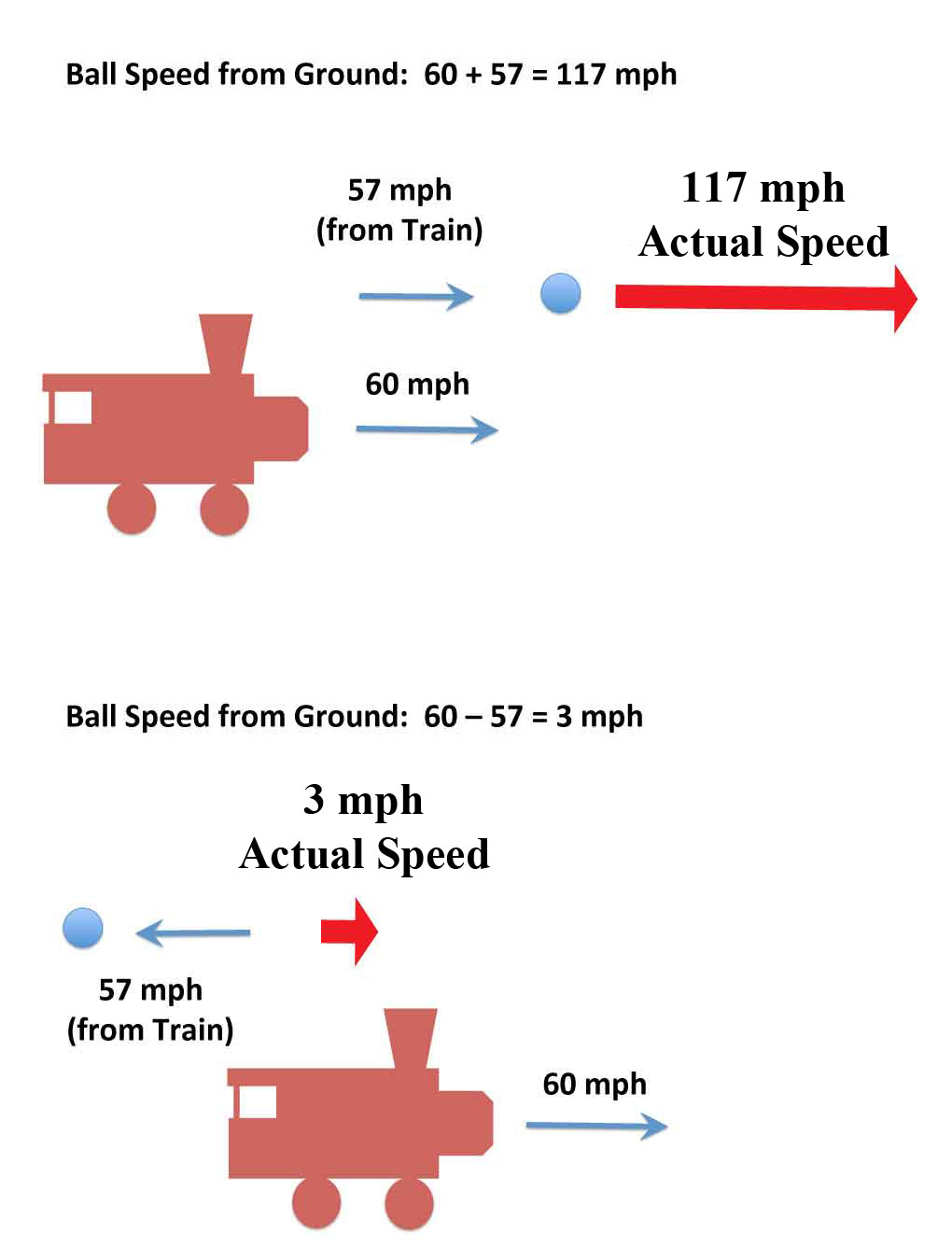
Relative Speeds

But now toss the ball from the caboose in opposite direction. The ball will now only move at 60 - 57 = 3 miles per hours.

So if you can measure the speed of the balls, you can calculate the speed of the train. If S_f is the speed of the forward ball and S_b is the speed of the ball tossed backwards, you can calculate the speed of the train as:

Train Speed = ^{(S_f - S_b)} / ₂

Albert thought a moment. If he shone a beam of light in the direction the Earth was traveling, then the light should act like the baseballs. That is, the beam would pick up the extra speed from the Earth.

Since we know the Earth was orbiting the Sun at 18.5 miles per second, the light will now be traveling at:

186282.3971 mps + 18.5 mps = 185370.8931 miles per second

But shine the light in the opposite direction the Earth moves and the beam will be traveling at:

186282.3971 mps - 18.5 mps = 185263.8931 miles per second

The difference between the forward and backward light is therefore

Difference = 185370.8931 mps - 185263.8931 mps

= 37 miles per second

That's 133,200 miles per hour! And this is the minimum difference. The solar system is also traveling at a certain speed. So the difference in the light beams will be even greater. Measuring the speed of the Earth should be a snap.

There was just a wee bit of a problem.

 

The Problem

And the problem is ...?

Well, the problem is if you want to see the difference in the light beams, you would have to get off the Earth and measure the speed of light independent of the Earth's motion.

Or look at it this way. If a ball is traveling 117 miles per hour and you're traveling 60 miles per hour in the same direction, then it looks like the ball is traveling 57 miles per hour in front of you.

But if the ball is traveling three miles an hour and you're traveling 60 miles per hour, it looks to you like the ball is traveling 57 miles per hour in the opposite direction. So you can't see a difference in the speed of the baseballs unless you get off the train. The same is true for light. From the Earth you couldn't detect any change in speed.

But what, Albert wondered, would happen if you have a mirror off at a right angle to the Earth and one in front of the earth?

Well, if you and everything else stands still - or rather you're standing still relative to the mirrors - the beams just go to the mirrors and bounce off.

 

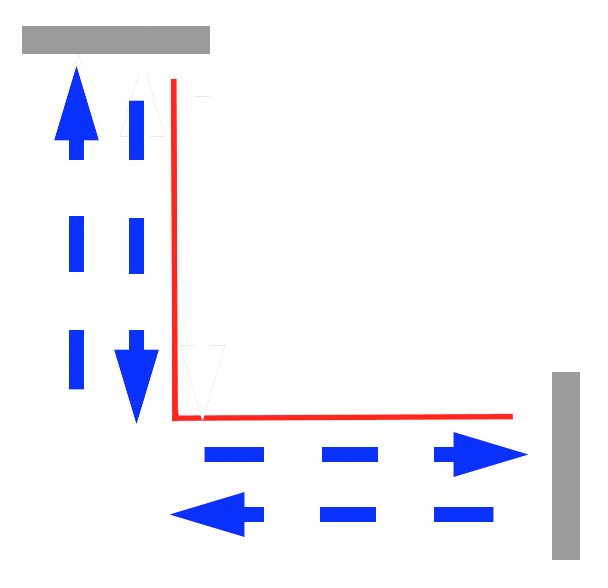
Albert's Experiment - General Plan

But if you step off the Earth and can watch the apparatus travel along, things would look different?

 

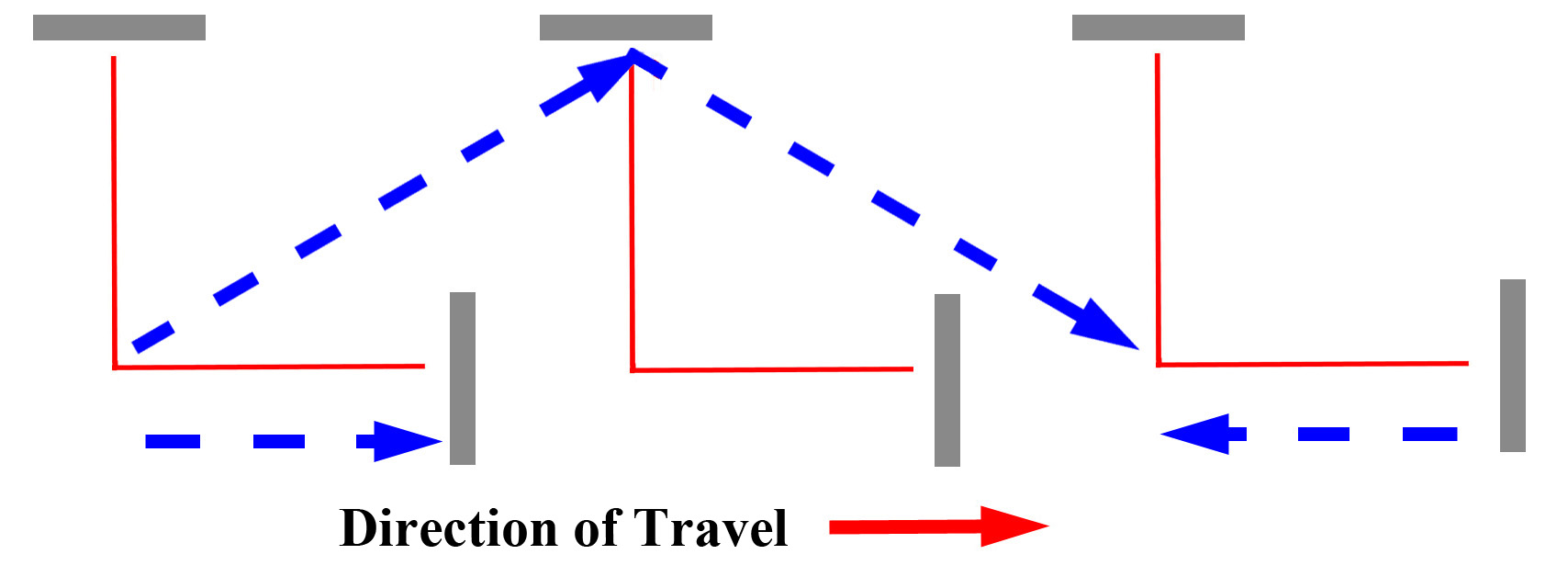
Albert's Experiment - General Plan

In that case you see the beams' motion as a combination of the up and down motion of the light bouncing off the two mirrors plus the forward motion of the Earth itself. So it looks like the beam that goes off at a right angle has a longer path.

But we can't still get off the Earth! So what can we do?

But remember, Albert was an expert in optics. He knew that light was a wave.

Waves have high points and low points. And if you bring two waves together, the high points might line up. In that case, the light will be brighter than either of the original beams.

But if the light beams meet so the high parts overlap with the low points, then the light beams cancel out. Ergo, you get no light.

 

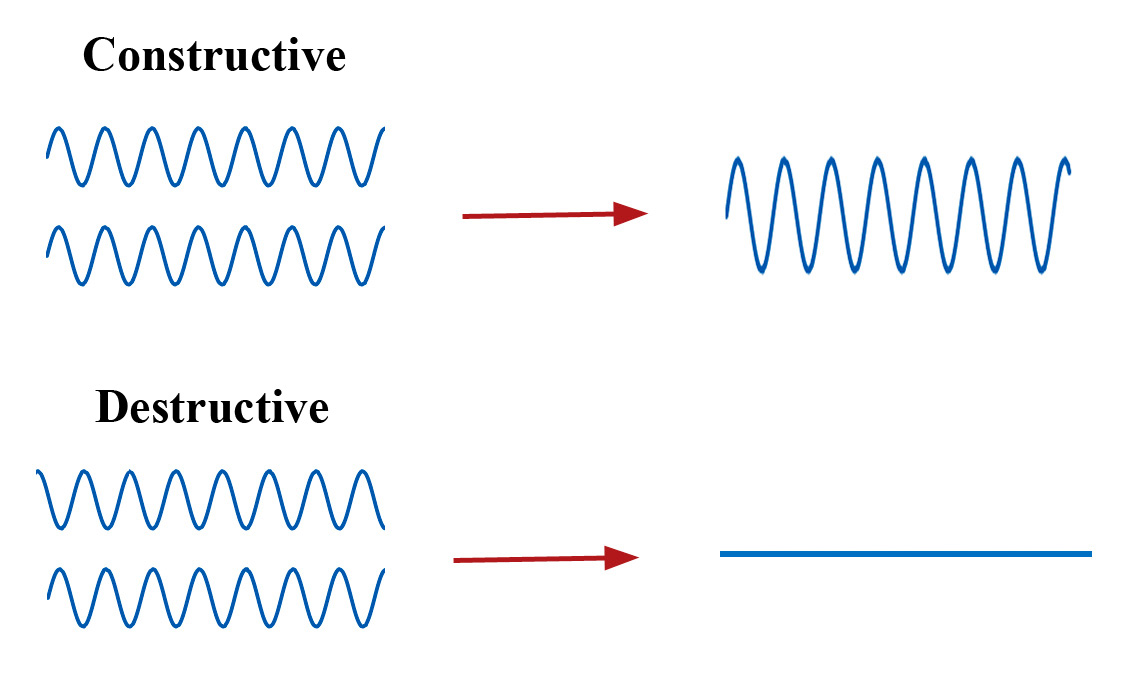
Interference: Constructive and Destructive

Light beams that interfere in a constructive manner result in constructive interference. If the light beams interfere in the destructive manner, it's called destructive interference.

OK, consider what happens when you shine a beam of light through two holes in a screen - this is called a double slit experiment. The light will, of course, spread out. So you have a bunch of light rays which all head toward the screen but they travel different distances to reach the screen.

Since they travel different distances, it means in some places the interference is constructive - producing a bright spot - or destructive - producing dark spots.

What you get, then, are a series of light and dark bands or rings - an interference pattern. And where the light and dark bands show up on the screen, depends on how far the holes are spaced, the wavelength of the light, and the distance the light travels.

 

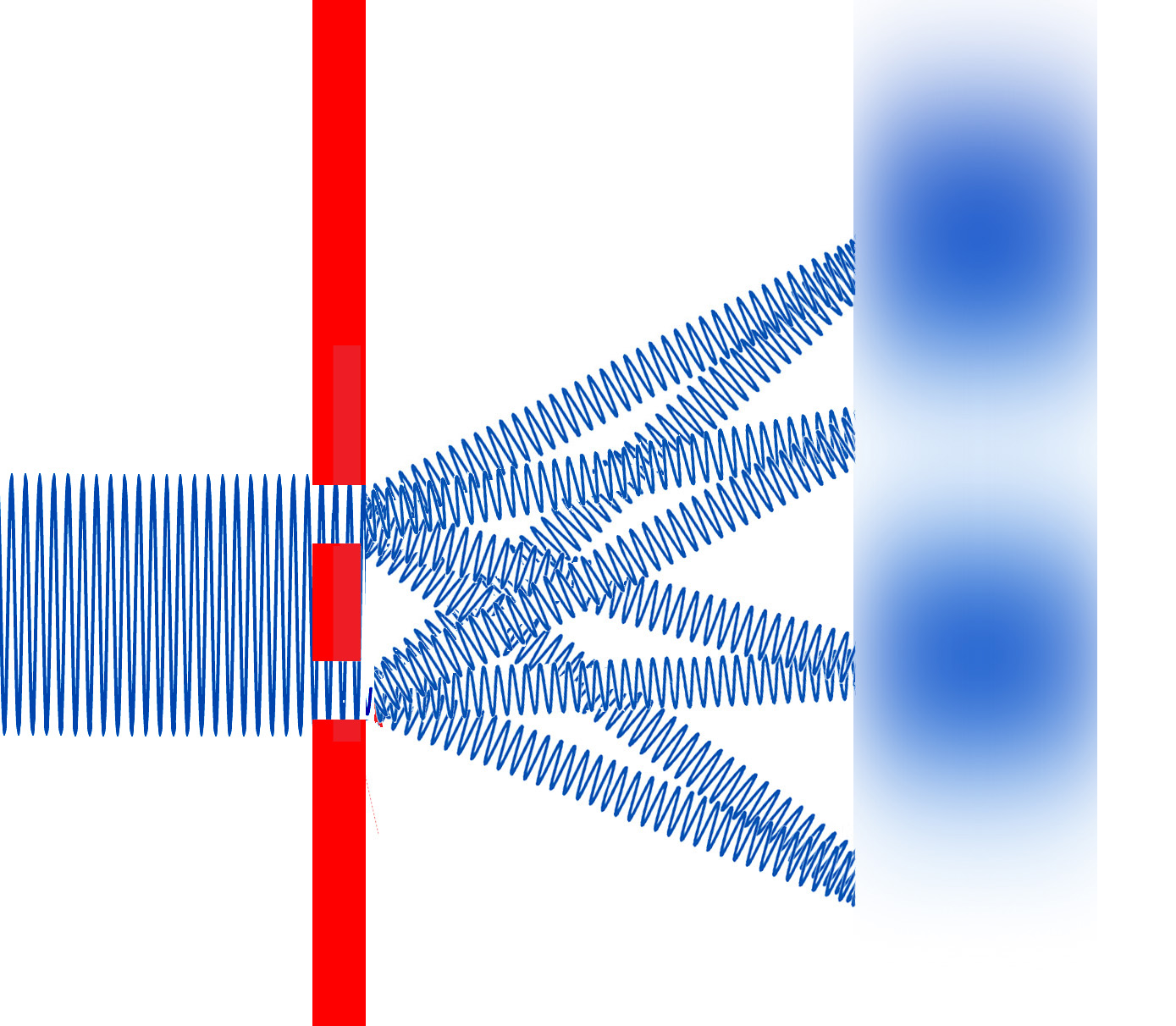
Waves and Interference

Another way to understand the interference pattern is by the way wave fronts meet. The high and low points expand out in circles from the sources of the light and they reinforce and cancel out to form the pattern which seems to originate from the point between the two light sources.

 

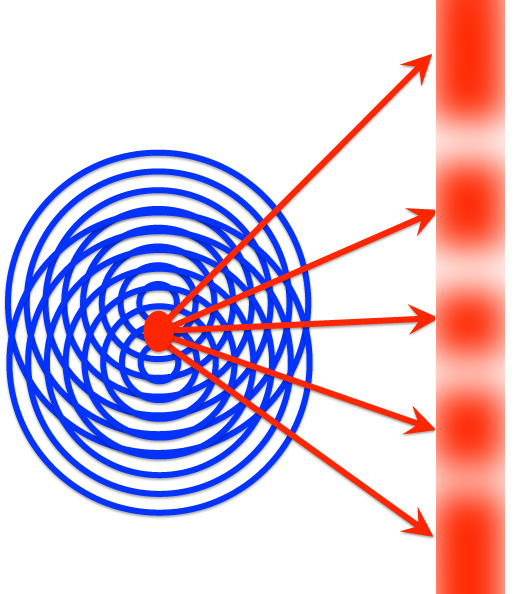
Wave Front Interference

Actually you don't need the double slits to get an interference pattern. Instead you also get interference patterns if you simply combine two light beams and send them through a slit or a lens. The beams combine, the light spreads out, and you get an interference pattern.

Knowing he didn't need the double slits for a happy experiment, Albert developed a plan. After all, he knew if you were out of slits, you were not out of cheer.

 

Albert's Gadget

What Albert did was to construct an apparatus now called the Michelson Interferometer. It had two mirrors mounted on two arms. One mirror is placed directly in the beam of a light source. The other is placed off at a right angle.

Next in the middle of the apparatus, Albert placed a half-mirror at a 45 degree angle to the beam of light. A half-mirror is a mirror where only half of the surface area is reflective. These are made by various ways. Some are made by sputtering the metal onto the glass plate but only enough to randomly cover half the surface area. You can also make a half-mirror by coating the mirror and then scraping off bits of the silvering here and there until half is coated and half isn't.

So when the light beam hits the half-mirror, half the beam goes straight through the mirror and the other half gets bounced off at a right angle. Both these beams hit one of the other mirrors, return to the half-mirror, and recombine. They then continue on to a screen and form the interference or the fringe pattern.

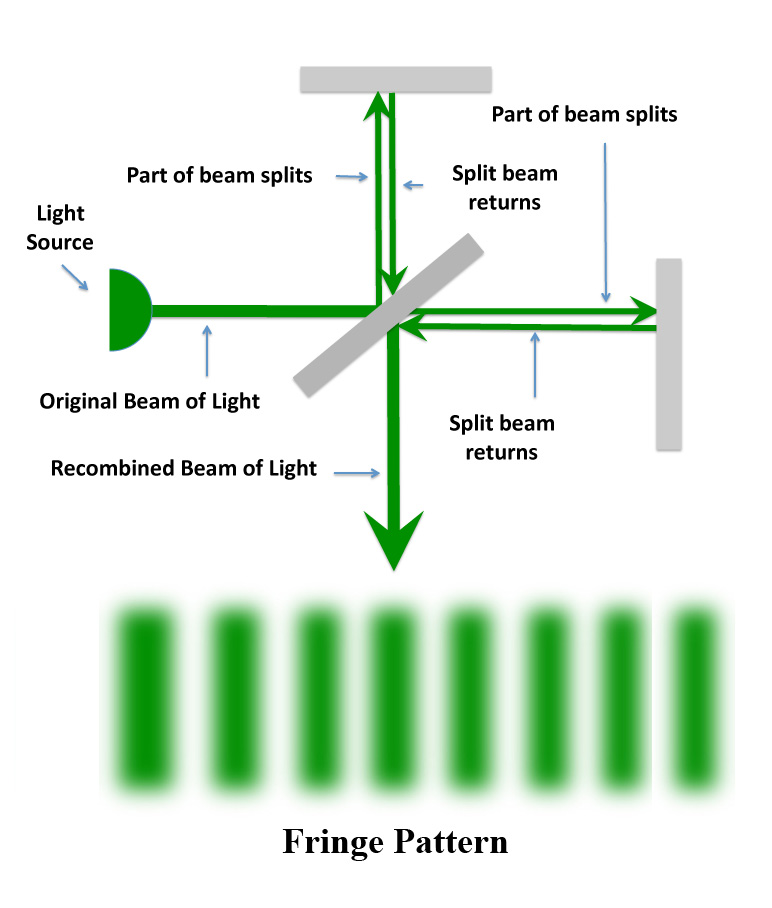
The Apparatus

OK. The combined beams form the interference pattern. But how does that help us measure the speed of the Earth through the ether?

Well, the positions of the bands of an interference pattern are extremely sensitive to the changes in the path lengths of the light. Even path differences caused by vibrations would change the lengths of the light beams and effect the position of the fringes. The device was so sensitive that if someone jumped on the pavement 100 meters away - essentially a football field length - the fringes disappeared entirely!

To avoid the problem of vibration, Albert and his colleague, Edward Morley, mounted the apparatus on a block of stone that was floating in a pan of mercury^**. The mercury, being a liquid, would form a level surface and it would also absorb vibrations. Albert could then smoothly rotate the apparatus. The changes in the fringes would show if the path lengths of the light were changing.

The experiment - called the Michelson-Morely Experiment - would easily detect even the minimum shift based on the speed of the Earth in it's orbit. The fringes would shift about 16 %. As we see, that's small, but definitely enough to measure.

But to repeat, 16 % is the shift you get just from the motion of the Earth round the sun. Albert and Edward were expecting a lot more. And then they could calculate the actual speed of earth moving through the ether - that is, through space.

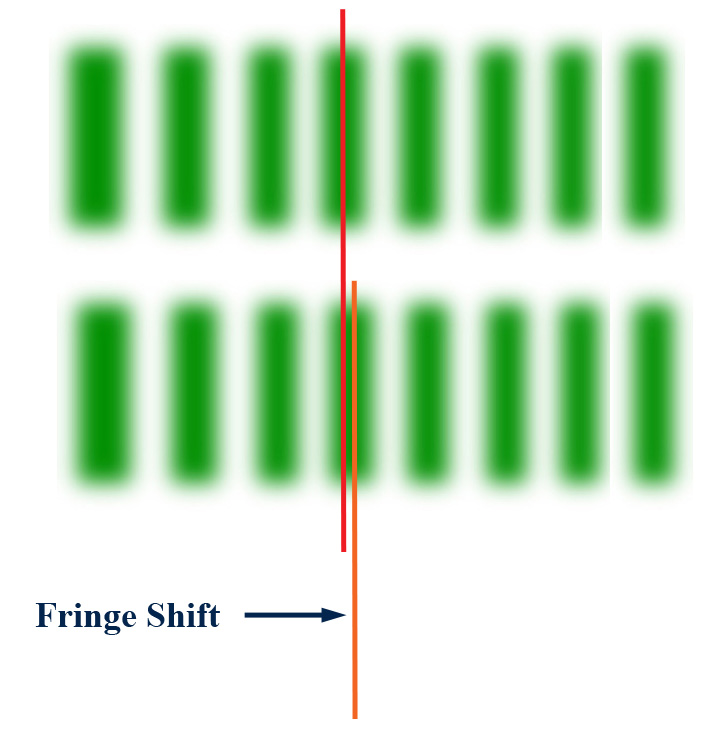
The Fringe

 

A Minor Problem

Well, humming merrily, Albert and Edward ran the experiment. And they were flummoxed by what they saw.

The shift wasn't 16 %. It was more like 2 or 3 %.

Well, you say, that just means the Earth was traveling through the ether slower than we thought. But remember, the Earth's minimum speed is about 18 miles per second. The shift had to be at least 16 %.

What was worse - and Albert deliberately pointed this out - the 2 % shift was simply experimental uncertainty. That is if you just rotated the interferometer all the way around to the starting place you'd see the fringes shift 2 % just by random chance.

In other words, Albert's experiment failed!

 

"Dr. Michelson! What Does It All Mean?"

Fortunately Albert didn't simply toss the data out. He wrote it up and published it. Later Albert and Edward refined their experiment. But no matter how careful they were, they saw no change in the speed of light.

One explanation, of course, is that the Earth is the center of the universe. That is, it is fixed and immovable. If it's not moving through the ether, then you won't get any change in light paths.

But to paraphrase Arlo Guthrie, that isn't very likely and we don't expect it. After all, if the Earth is the center of the universe, how can it circle the Sun?

Now there is one philosophy of science that says you can always keep any theory if you are willing to make it more and more complex. For instance, you don't need to have a Sun centered solar system.

You can for instance, simply put the Sun circling the Earth. Then you have the other planets circle the Sun. In fact, that is exactly what the famous Danish astronomer Tycho Brahe - who didn't buy into Copernicus - did.

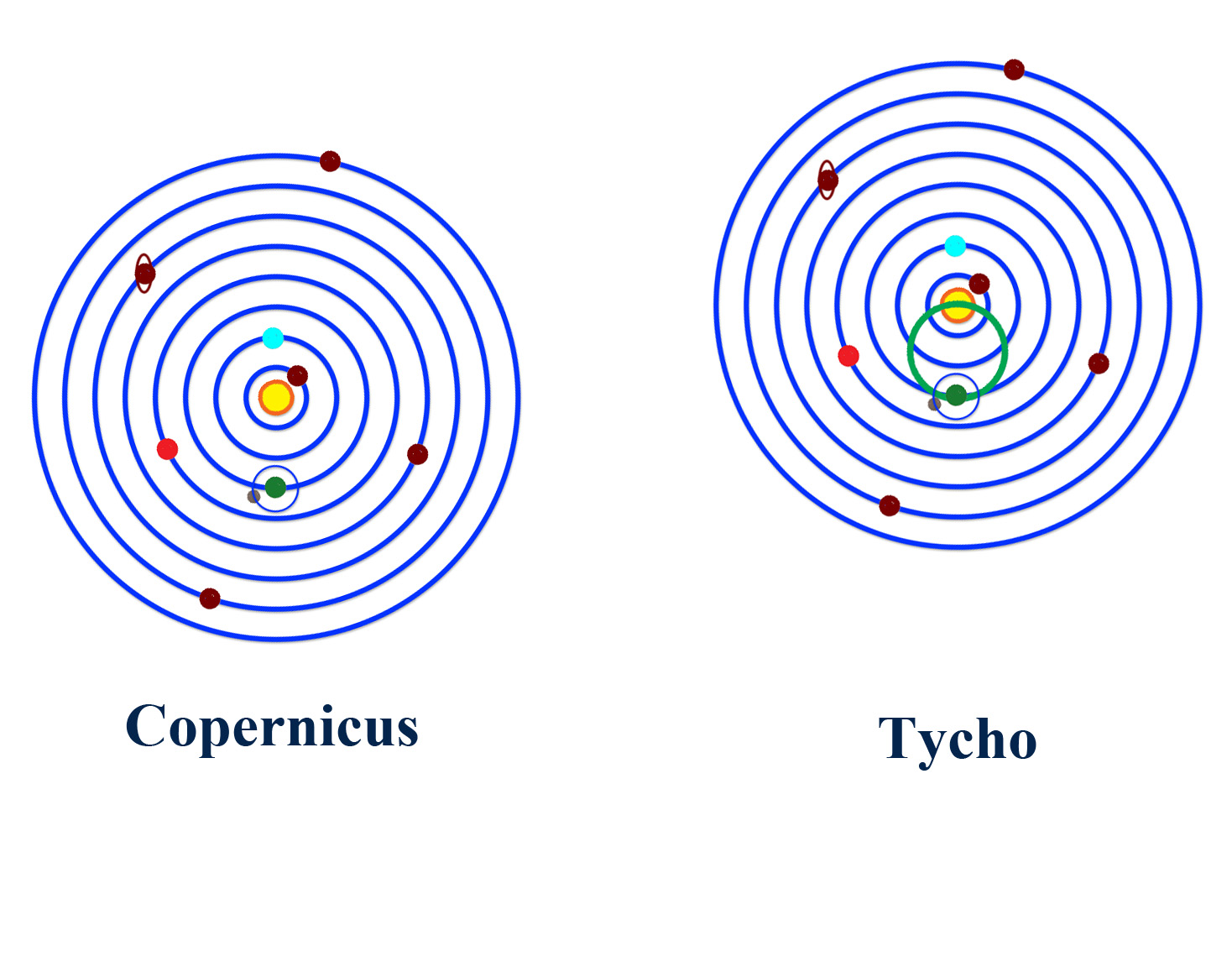
"Keep It Complex, Stupid!" - Tycho Brahe

What Tycho's system did not do, though, was explain the way the angle at which a star's light changed as it hit the earth during different times so the year. This was due to stellar aberration - not to be confused with stellar parallax. It was stellar aberration that James Bradley used to measure the speed of light and it is an effect that is far, far easier to explain if the earth is circling around the sun.

 

Keeping the Ether

But getting back to the ether, one explanation for Albert and Edward's unexpected findings - no change of the speed of light occurred - was proposed by the German physicist, Hendrik Lorentz.

The ether, Hendrik suggested, is squashing the Earth and everything on it. Since your measuring device is also squashed, it looks like the speed hasn't changed.

For instance, say a car drives by and you measure the distance it travels in an hour - and you use a one foot ruler. You find that the car traveled 264,000 feet. So the speed was 50 miles per hour.

But now the car drives by at 25 miles per hour. So it would travel only 132,000 feet in an hour.

But what if your one foot ruler shrinks to half a foot and all the markings are reduced accordingly? You'd still measure 264,000 feet and calculate the car driving at 50 miles per hour.

 

The L-Factor

Being good at math, Hendrik came up with an equation for how much everything would have to be squashed to explain Albert's experiments. You simply multiply the length that you measure by the famous Lorentz factor.

√1 - (^v/_c)²)

where v is the speed you are traveling through the ether, and c is the speed of light.

So to calculate the squashed distance, you use the formula:

l^' = l × √1 - (^v/_c)²)

where l is the "real" length of the object, c is the speed of light, v is the speed the object is traveling, and l^' length of the object after the ether squashes it.

How much will the measuring stick get squashed using the Lorentz factor? Well, say you have a stick that is 1.000000000000000 meters long. Now hold it in the direction the Earth is traveling. If the earth is traveling at 18.5 miles per second, it will shrink to a length of:

Length = 1.000000000000000 meter × √1 - (^18.5 / _186282.3971)²)

= 0.999999995068605 meters

In other words, the meters stick gets squashed by 0.000000004931395 meters or 0.000000000125257 inches, and everything else is squashed in proportion. If this happens, then the measured speed of the light beams wouldn't change. Hendrik also developed some more equations using his factor that explained other effects of the ether.

 

Disposing of the Ether

The trouble was as scientists did increasingly complex and exact experiments, the ether theory began to have less and less appeal. The ether had to be some kind of fluid - a gas or a liquid - but it couldn't be detected. It had no mass, and it would be so thin that you couldn't feel it even if you sped through it at 18 miles per second.

At the same time, the ether had to be firm enough to squash a planet and everything on it. It also had to snap back and forth with incredible rapidity to transmit electromagnetic radiation. So by the end of the 19th century, the ether was looking more and more like an impossible deus ex machina to explain why light didn't change speed.

 

"Time, Gentlemen! Please!"

We now turn to another Albert - Albert Einstein.

Since 1902 Albert had been working as a technical assistant at the Swiss Patent Office. He had a teaching degree from Zürich Polytechnic in math and physics but hadn't been able to land a job. So he applied for the patent job and got it.

At the Patent Office, the pay was pretty good - 3500 Swiss francs a year ($2333 in US dollars or about $63,000 in today's money). Albert was also popular with his co-workers, and in what has long vanished from the workplace, he even liked and respected his boss.

Best of all the work was not physically taxing. So after the day's work was done, Albert could spend his off-hours working on physics problems. That was a good thing since while Albert was at the Patent Office, he was also working toward his Ph. D. at the University of Zurich.

Although today someone getting a Ph. D. while working at a full time job isn't unheard of, it's certainly not common. And people wonder just how the heck Albert 1) got a Ph. D. while being a patent examiner, and 2) developed a theory that is considered one of the most important in the history of science.

We need to remember that in Albert's day that universities had begun groping their way from being affectations for rich kids to institutions that trained students to make a living. Standards were evolving, and what is surprising is how much flexibility a student had once they got in.

For one thing in European universities, there were no exams given during the actual classes. No attendance was taken, and no homework collected.

Instead, the tests came at the end of the term. Everyone took the same tests no matter whose lectures you attended. For some curricula, the exam questions were given in advance, and if you didn't pass the test you could take it again.

It's no surprise, then, that good students could almost be cavalier in their studies. Albert Schweitzer seems to have spent his time at the University of Straßburg more or less reading on his own with minimal input of his professors. Ludwig Wittgenstein submitted a thesis to Cambridge University that was a booklet written while he was a prisoner of war in Italy (naturally it helped that the evaluation "committee" were two of his personal friends, Bertrand Russell and George Moore). Even today the Doctor of Science is awarded on the basis of published research and you don't take extra classes or submit an actual thesis.

Albert was in fact a good student - the idea that he had poor grades is hogwash - and from 1901 to 1904 he published five papers. If he couldn't make a lecture he was able to get the notes from his fellow students and he was able to pass all exams and write a thesis. In 1909 he got his doctorate.

But it was in 1905 - while he was at the patent office and at the University of Zurich - that Albert wrote three papers, and they made a pretty big splash in the scientific world.

One of Albert's papers explained "Brownian motion" - that is, the way tiny particles in a liquid - like pollen grains in water - bounce around. Albert showed the motion was from the statistical bombardment of molecules against the grains. This was the first real indication that atoms and molecules had a real existence and were not just mathematical conveniences.

In another paper he explained the photoelectric effect - why some metals emit electricity when light shines on them. To do so, Albert used the new idea that light is quantized into discrete energy packets. Both of these papers were so important that they could have - and this last one did - land him a Nobel Prize.

But in a third article Albert offered his explanation to the conundrum of why light didn't change velocity in Albert (Michelson)'s experiment. The solution was indeed very simple. That's just the way it is.

 

Back to Fundamentals

You'll sometimes read on the Fount of All Knowledge that Albert wasn't well known to the general public until 1945 after the atomic bombs were dropped on Hiroshima and Nagasaki. And you'll also read that only 12 (or 10 or 20) people can understand the Theory of Relativity.

Neither statement is true. Albert first came to the general public's attention around 1920 with some high-profile stories about Relativity. We also saw that he was well enough known to be denounced by a major American public figure in 1929. In 1933, the New Yorker Magazine ran what no doubt passed for a trendy and witty "profile" about Albert, and by the late 1930's Life Magazine had published articles about how famous Albert and his theories were.

And although not every Joe or Josephine Blow on the street understood the nitty-gritty of Albert's theories, all physicists did. But any Joe and Josephine Blow who had a middling amount of algebra could have learned about it as well.

What Albert did was start from a simple premise based on the Michelson-Morley Experiment. The speed of light, Albert proposed, did not travel through any ether. It was just that the velocity of light as measured is a fundamental constant of nature.

At first this last statement may not seem a big deal. After all, if you measure the speed of light and you're careful, everyone should get the same speed.

But there's more to it. For instance, if the source of the light beam is a spaceship traveling at 50 % the speed of light past a planet, then "classical" physics would have the person on the planet see the light beam traveling at the speed of light, 186,000 miles per second plus the speed of the spaceship or 93,000 miles per second. But someone on the spaceship measuring the same beam of light, should only see the light travel at 186,000 miles per second - the "normal" speed of light.

Instead Albert was saying that both observers will see the same beam of light travel at the same speed, that is, 186,000 miles per second. Remember that this just wasn't a crazy theory. The experimental data backed this up completely.

So what, Mr. Natural - or rather Dr. Einstein - does it all mean?

Well, if you take Albert (Einstein)'s very simple principle, you find a lot of interesting things.

For one thing you find out that as you speed up, time itself slows down. But it doesn't seem to you that your time slows down. But to someone who isn't going as fast as you are, your time slows down.

Let us elaborate.

Now Joe Blow is on a Spaceship, and he keeps time with a light clock. A light clock is simply a beam of light bouncing between two mirrors. We'll designate the time it takes for the beam to go from one mirror to the other and back as a "Spaceship second" although it's obviously much faster than that.

The Light Clock

But suppose the space ship is now flying by the Earth at some superfast speed. And someone on Earth looks up with a telescope and follows the beam of light.

What does the Earth-bound observer see? Well, he will see the light beam travel further than Joe Blow on the Spaceship did.

That's because the light beam doesn't go straight up and down when seen from Earth. Because the Spaceship is moving, the light follows a zig-zag path.

Someone on Earth Looks Up

Now consider this. If the light as measured on the spaceship travels a shorter distance than as seen by the observer on Earth, but both see the light traveling at the same speed, then time must be slower on the spaceship as measured on earth.

We easily can prove this with middle school math and in doing so derive an equation for what is called time dilation. To do this we look at the distance traveled by the light from both the Spaceship reference frame and the Earth reference frame. It's also simpler if we just look at the time it takes the light to travel from one mirror to the other rather than the round trip.

Remember that distance you travel is simply your speed (velocity) multiplied by the time you travel.

distance = velocity × time

At first we'll consider the time as seen from the Earth, and call it t₁. The distance the Spaceship travels is therefore d₁.

d₁ = v × t₁

where of course v is the velocity of the Spaceship.

Now the distance the light travels as measured on the Spaceship is the speed of light, c, times the time it takes the light to travel between the mirrors - but only the vertical distance. Call that distance, d₂, and the time as measured on the space ship we'll call t₂.

d₂ = c × t₂

But how far does the Earth observer see the light travel? Well, it's the speed of light, c, multiplied by the time it takes for the observer on Earth to see the light pass between the two mirrors. Now a little reflection will let us realize this time happens to be our earlier time, t₁. We'll call the new distance d₃.

d₃ = c × t₁

And all of these distances, speeds, and times, form a right triangle:

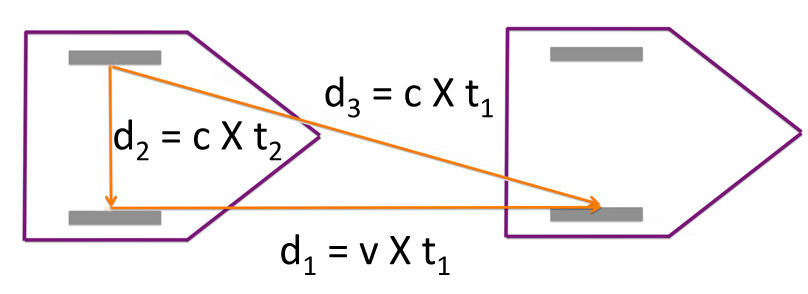
Space Clock: From One Frame to Another

And are related by the Pythagorean Theorem:

d₁² + d₂² = d₃²

which is the same as:

(vt₁)² + (ct₂)² = (ct₁)²

And mit ein bischen Mittelschule Algebra:

(vt₁)² + (ct₂)² = (ct₁)²
v²t₁² + c²t₂² = c²t₁²
c²t₂² = c²t₁² - v²t₁²
c²t₂² = t₁²(c² - v²)
t₂² = ^{t₁2(c2 - v2)} / _c²
t₂² = t₁²(1 - ^v2/_c²)

And we get the final formula:

t₂ = t₁√1 - (^v / _c)²)

 

The Equivalent Equations of Albert and Hendrik

Notice that Albert's equation for time looks an awful lot like the equation Hendrik calculated for length.

l₂ = l₁√1 - (^v / _c)²)

Hendrik

 

t₂ = t₁√1 - (^v / _c)²)

Albert

So the Lorentz Factor arises from the principle that the speed of light is the same regardless of reference frame and simple middle school algebra.

Now for a little known fact. Hendrik had also worked out the equation on time. And a lot more.

And the truth is the equations that Albert used in his Special Theory of Relativity were those developed by Hendrik. So why, we wonder, does Albert get all the credit?

Actually the slighting of Hendrik actually came later. At first people called Einstein's Special Theory of Relativity, the Einstein-Lorentz Theory of Relativity.

Although today you'll read how some people rant that Albert "plagiarized" his equations from Hendrik, it was simply that today's rigorous standards of citation were neither expected nor needed. All physicists knew the equations were from Hendrik's early papers. For his part, Hendrik praised Albert's theory, and Albert said he thought Lorentz was one of the greatest physicists of all time. So if it doesn't bother them, it shouldn't bother us.

 

Now the Paradox

What Albert and Hendrik were able to show was that this Special Theory of Relativity had other strange conclusions. As you speed up, length not only contracts and time slows down, but Albert even showed that mass increases. All these changes are by multiplying or dividing by the Lorentz factor √1 - (^v/_c)²).

But some things a lot of people didn't like. For instance, if you had two Spaceships traveling past each other, you conclude both people see the other as moving slower. Is this possible?

Impossible, said some. Relativity, they said, can't be true. Case closed.

On the other hand, remember how we got Relativity. We started with an observed experimental result that has been repeatedly confirmed. Then we use simple and proper reasoning using - and pardon us again if we shout - NOTHING MORE COMPLEX THAN MIDDLE SCHOOL ALGEBRA! So rather than dismissing either experimental facts and middle school math, perhaps we should dig a little deeper.

 

The Twin Paradox

Now we come to what causes many people trouble - and believe it not, we also mean it bothered some famous scientists. We are referring to one of the most famous "thought experiments" in science.

That's the Twin Paradox.

Suppose you have two twins. One gets in a Spaceship and the other stays on Earth. Let's say the Earth Twin sees the Spaceship head off at 185,000 miles per second - that is nearly the speed of light. By Earth's time, the Spaceship travels 25 years (measured on Earth), turns around, and comes back. So he spent 50 years in space.

But in the moving Spaceship, the time is calculated with Special Relativity. So we get:

Trip Time (on Spaceship) = 50 years × √1 - (^185,000/_186,000)²)

= 50 years × √1 - 0.989276217

=50 years × √0.010723783

=50 years × 0.1035557

= 5.2 years.

So if the Twins were 25 years old at the start of the trip, the Earth-Twin is now 75 years old. But his Twin - who flew in the rocket - is only 30 years old!

But here is the problem:

If there are no absolute reference frames, the Space Twin could consider himself to be stationary. In that case it is the Earth that is moving off at 185,000 miles per second. So the Earth returns after a total of 50 years. So it is the Earth Twin who has aged five years!

Now we said time is not an absolute in Relativity. But that does not mean that a person can be 30 years old and 75 years old at the same time. So we seem to have found a true contradiction. And contradictions, the mathematicians tell us, refute the premise.

Well, when some people read this, they conclude relativity is hokum, poppycock, and hogwash.

But hold on there, pilgrim. Not so fast.

Remember that Relativity is a simple mathematical consequence from an experimental observation. Not only an experimental observation, but an experimental observation that has been repeatedly verified by independent methods.

So how do you resolve the Twin Paradox? Well, there's a number of ways you'll read on the Fount of All Knowledge (the Internet) - and sometimes even in those non-electronic devices with white flappy things in the middle (can't remember what they were called).

The first way out is to say that the Twin Paradox is not a valid thought experiment. Remember, Special Relativity is limited to motion traveling in a straight line and at a constant speed. So you can't use special Relativity when talking about a round trip.

Another way to explain the paradox is to say that it is the person who moves relative to the inertial reference frame and who undergoes acceleration that gets younger. So the Spaceship leaves, heads off, turns around, and comes back, leaving the reference frame and undergoing acceleration. The Space Twin is the younger. Note this - quote - "explanation" - unquote - doesn't really explain anything. It just restates the observation.

There is also a popular approach where you just smirk at the question and say, well, you need to learn about Albert's General Theory of Relativity. So just learn tensor analysis, take advanced physics courses, and you'll understand.

The truth, though, is if you keep track of everything and just use the Lorentz factor and middle school math - everything works out.

 

Reference Frame 1: The Frame Where the Earth Stood Still

First we must emphasize, actual physical reality must be consistent. The Twins can't both get younger and older. Reality is reality.

So we have to ask: Is it possible for the two twins to agree that the Earth Twin is older and the Space Twin is younger regardless of who we consider moving? The answer is yes.

First we start out with the Earth standing still.

To keep track of the distance, we will have the Spaceship travel to a Planet. We will put this Planet 6 light-years from the Earth (for the real beginners, a light-year is the distance light travels in one year which we know is exactly 5,874,601,673,407 and ³/₁₀ miles).

What we're going to do next is to let the two Twins look at each others' clock. Of course, the clocks have to be viewed on a television set, and the images are sent via radio waves. The radio waves travel, of course, at the speed of light.

We also need to keep in mind the three points of Special Relativity.

First, time slows down for an object moving relative to another when measured from the "stationary" reference frame.

Second, length contracts for an object moving relative to another, again when measured from the stationary reference.

Third, adjustments are made using the Lorentz factor, √1 - (^v/_c)².

To keep the calculations simple, we'll keep with numbers used by a well-known physicist when talking about Relativity. That's to let the distance the Space Twin travels be 6 light-years and also to let the Spaceship travel at 111,699 miles per second. That's 60 % the speed of light. This is 0.6 light-years per year which astronomers and physicists write as 0.6c. Light, of course, travels at one light year-per year or 1c.

Now, as the psychiatrist said, we can begin, yes?

 

Gentlemen, Start Your Engines!

So what we have now is the Space Twin taking off in the Spaceship and head toward the Planet. But he can watch the clock on Earth on his television.

Similarly the Earth Twin remains on Earth but can watch the Spaceship and monitor the Spaceship's clock on his television.

So the Earth Twin watches the Space Twin set out for the Planet 6 light-years from Earth. And the velocity is 0.6c.

So as measured on Earth, it takes the Space Twin:

^{6 light-years} / _{0.6 light-years per year} = 10 years

to make the outbound trip.

And does the Space Twin's trip also take 10 light-years?

Nope. Remember the Space Twin's Clock slows down by the Lorentz factor:

Time for Space Twin = Time for Earth Twin × √1 - (^v/_c)²)

So plug in the values and we get:

Time for Space Twin = 10 years × √1 - (^0.6c/_c)²)

which is

Time for Space Twin = 10 years × √1 - 0.6²)

and becomes:

Time for Space Twin = 10 × √1 - 0.36)

= 10 × √0.64

= 10 × 0.8

= 8 years.

So here the Lorentz factor is 0.8, and the Space Twin's clock will read 8 years when it reaches the planet, not 10. But at that time, the Earth clock will still read 10 years.

But it is important to remember that the Space Clock really is reading 8 years. If both the Space Twin and the Earth Twin are watching the Space Clock, they both agree that the Space Clock reads 8 years when the Spaceship reached the planet.

[Note: The Earth Twin does not see the Space Twin reach the planet after 10 years because there is a lag of the light and radio waves. It is an interesting exercise to figure out what and when the Twins see, but we'll pass that for now.]

And on the trip back?

Again the Earth Twin sees the Space Twin's clock go from 8 years to 16 years while his own clock goes from 10 years to 20 years. Similarly the Space Twin watches the Earth clock go from 10 to 20 years while his goes form 8 to 16 years.

So to summarize everything so far:

The Earth Twin sees his clock run from 0 to 10 years when the Spaceship reaches the Planet. And he agrees the Space Twin's clock ran from 0 to 8 years.

The Space Twin sees his clock run from 0 to 8 years when he reaches the Planet. And he agrees the Space Twin's clock ran from 0 to 10 years.

Both agree the return trip takes the same amount of time as the outward trip.

So both agree the Earth Twin aged 20 years for the round trip and the Space Twin aged 10 years.

Finally, everyone agrees the Space Twin is four years younger when he gets back to Earth.

And now ....?

 

Reference Frame 2: The Day the Spaceship Stood Still

Now at this point, a lot of the Explainers of the Twin Paradox stop and say, see, everything works out. But stopping here is what causes the problems.

That's because the Doubters say, hold on. You only stuck with the stationary Earth-Planet reference frame. You have to prove that Relativity works if the Spaceship stays stationary and the Earth moves off.

And as we mentioned there are various ways to wave your hands and dismiss the topic. You can also use some fancy pants Space-Time diagrams and such stuff. But you rarely get a simple middle school algebra explanation like we saw for the Earth reference frame. This neglect is what has led even some famous scientists to say the two reference frames are irreconcilable and the theory is wrong.

For instance, not only do the Doubters point out the Twin Paradox requires the Twins to be both old and young at the same time, they reason thusly. If the Earth-Planet system is moving at 0.6c, then by the Lorentz factor being 0.8, the 6 light-year distance contracts to 4.8 light-years.

But for the Earth Twin to age 20 years overall, he must travel 6 light years on each leg of the trip, not 4.8!

Explain that, the Doubters say.

Well, it turns out we can. But we need to use another finding of Relativity.

That's the Relativity of Simultaneity.

 

The More Things are Simultaneous ...

At this point you might go into spittle-flinging diatribes.

Now we're introducing another odd-ball finding of Relativity?

What is this? Just another way to make the numbers balance out?

Well, not quite. The Relativity of Simultaneity is another inevitable conclusion of the speed of light being the same regardless of reference frame and middle school algebra. Then we'll find out that if two things happen at the same time in one reference frame, they do not happen at the same time in another reference frame.

Now in the simplest introductions of Relativity, you almost never read about the Relativity of Simultaneity. But there's really no reason to omit what is as important as the other unusual findings of the theory. True, there are a few more steps in the math, making the bookkeeping a bit more involved. But it's all easy enough as we will see.

Consider the following. We'll put a beacon on the Spaceship. The beacon can at any time emit a flash of light. And on the Earth and the Planet, there are detectors to determine when the light reaches them.

Remember, the speed of the same beam of light will be measured traveling at the same velocity in both reference frames.

Now we ask, when do the Space Twin and the Earth Twin see the light reach the detectors?

It would seem that if you flash the beacon at the halfway point, the beams of light should reach the Planet and the Earth at the same time.

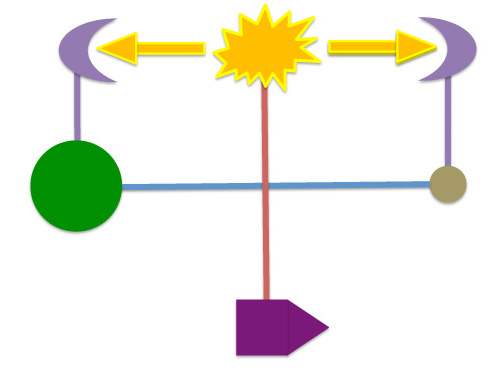
Simultaneous Events?

Is this what happens? Well, yes and no.

First let's look at the Spaceship as seen from the Earth-Planet reference frame.

The Earth and Planet are standing still. The Spaceship takes off, moves to the halfway point, and then it flashes the beacon

So the beacon flashes when it is halfway between the Earth and Planet - 3 light-years from Earth. So by Earth's time the Spaceship has been traveling:

^{3 light-years}/_{0.6 light-years per year} = 5 years

We emphasize, the speed of light is a constant regardless of the reference frame. Light does not pick up the speed of the source of the light. And your speed does not change the apparent speed of the light. Repeat. The speed of light as measured is a constant.

So the beacon flashes and the two beams of light start off from the half-way point. One of the beams heads to Earth and the other heads to the Planet.

Both light beams travel (of course) at the speed of light for 3 light years. So the beams take 3 years to reach the Planet and the Earth.

From the Earth reference frame, then, the light hits the detectors at the same time. Counting from the beginning of the trip, that's in:

5 light years + 3 light years = 8 light years

And of course the Spaceship has to travel another two years to reach the Planet.

To see this experiment illustrated click on the image below.

 

Simultaneity: From the Earth

And from the Spaceship reference frame?

Well, from the Spaceship reference frame it's the Spaceship that is standing still. And the Earth-Planet "system" is moving. Since the Earth and Planet are moving, the distance between them contracts from the original 6 light-years to 4.8 light-years due to the Lorentz factor being 0.8. So the halfway point is 2.4 light-years.

Halfway Point for Space Twin = ^{6 light-years × 0.8 (Lorentz Factor)} / ₂

= 2.4 light-years.

          Stationary Spaceship / Moving Planets

Now here's where things get interesting.

Remember from the reference frame of the Spaceship, the planet is moving toward the rocket. And so the beam of light is not only moving toward the planet, but the planet is also moving toward the approaching beam of light. So the light beam will reach the planet quicker than if the Planet was not moving.

How much quicker is pretty easy to calculate. Just divide the distance - 2.4 light-years - by the sum of the two velocities.

That is, the time needed for the light to reach the Planet after the light flash is:

Time = ^{2.4 light-years}/_{(1+0.6) light-years per year}

= ^2.4/_1.6 light-years

= 1.5 years

And since the Planet has been traveling for 2.4 light-years before the beacon flashed, it was already traveling for:

^{2.4 light-years}/_{0.6 light-years per year} = 4 light-years

And the total time it takes the light to reach the Planet from the start of the trip is:

1.5 years + 4 years = 5.5 years.

Not the same as the 8 years in the Earth reference frame.

Now things get veeeerrrrrrrrryyyy interesting.

Remember that the Earth is also speeding away from the light source when the beacon flashed. So the light beam has to catch up with the Earth. So the time will be longer than if the Earth was standing still.

To find the time, then, we divide the distance by the difference of the speed of light and the speed of the Earth.

So after the beacon flashes, the Earth travels:

Time = ^{2.4 light-years}/_{(1-0.6) light-years per year}

= ^2.4/_0.4 years

= 6 light-years

And since the Earth has been moving for 4 years before the flash, the total time it takes the light to reach the Earth is:

4 years + 6 = 10 years.

So let's sum up what we've found.

Earth-Planet Reference Frame
(Spaceship is moving)

Time Light Reaches Earth: 8 years
Time Light Reaches Planet: 8 years

 

Spaceship Reference Frame
(Earth and Planet are moving)

Time Light Reaches Planet: 5.5 years
Time Light Reaches Earth: 10 years

 

Ha? (To quote Shakespeare)

So it's true!

In one reference frame the light beams hit the Planet and Earth at the same time.

And in another reference frame, they don't!

Yep. And to see this strange conclusion in an animation, click the image below.

Simultaneity: From the Spaceship

So this does indeed show us that events that are simultaneous in one reference frame are not simultaneous in another reference frame.

But if you watched carefully you might have noticed that as we calculated, the light reached the Earth in 10 years.

But the trip of the Planet to the Spaceship only took 5.5 years! And so if we use the Spaceship reference frame, the Earth kept going after the trip was over for the Planet!

But there's even something more unbelievable. You may have noticed that the Earth traveled 10 years - but the Planet only traveled for 8 years. But believe it or not, this isn't a problem.

In fact, this Relativity of Simultaneity will make things work out.

Finally.

 

Making Relativity Work

We do, though, have to do things a little different.

First of all we will suspend the beacon halfway between the Earth and the Planet. And it can be set off by either the Space Twin or the Earth Twin by sending a radio signal. Of course, the signal travels at the speed of light.

 

The New Arrangements: Beacons in Space!

As a simplification we'll only consider the outward leg of the trip realizing the return trip takes the same length of time.

What we will do is have the Earth delay sending the radio signal long enough so that when the beacon flashes the light beam will reach the Planet at the same time as the Spaceship reaches the planet.

First we'll use the Earth-Planet reference frame. That is, the Earth and the Planet are motionless, and the Spaceship is in motion.

Now what we do is to have the Earth Twin wait 4 years after the Space Twin takes off before he sends the signal. And remember the beacon sits halfway between the Earth and the Planet distance of 6 light-years. So the radio signal travels half the distance - 3 light-years - and sets off the flash.

So the light beam reaches the beacon and the beacon flashes:

Time = 4 years + 3 years

= 7 years

after the start of the trip.

And the light beam from the beacon must travel another 3 light-years to reach the Planet - or 3 more years. So after the Spaceship takes off, the light beam reaches the Planet in a total of:

Time = 7 years + 3 years

= 10 years

But remember. The Spaceship travels at 0.6c for 6 light-years. So it also reaches the Planet in:

^{6 light years} / _{0.6 light-years per year} = 10 years

So we are correct in our calculations. From the Earth-Planet reference frame, both the light beam and the Spaceship reach the Planet at the same time, after 10 years.

And when does the light reach the Earth?

Again the trip has been going on for 7 years before the beacon was flashed. And the light again travels for 3 more years.

So the light reaches the Earth in:

Time = 7 years + 3 years

= 10 years

So the two beams of light reach the Earth and the Planet at the same time and after 10 years. This agrees with our earlier calculation using the stationary Earth-Planet system. Of course, the return trip would also take 10 years for a round trip of 20 years.

Again we can see this scenario in action:

Trip Timed by the Beacon: From the Earth

To summarize so far, for a stationary Earth-Planet system and a moving Spaceship.

The Earth Twin sends the signal to the beacon after 4 years. The radio signal takes 3 years to reach the beacon and so the beacon flashes after 7 years.

The light reaches the Earth and Planet at the same time, after 10 years. This is also the time the Spaceship reaches the Planet.

The return trip takes the same length of time as the first leg of the trip.

Which brings us to the conclusion:

The Earth Twin sees the Spaceship return to Earth after a total of 20 years by the Earth clock.

Of course, if the Earth Twin were to monitor the Spaceship's clock, Space Twin would experience only 8 years for the first leg of the trip or 16 years for the round trip.. So we also conclude:

The Earth Twin determines the Space Twin is 4 years younger after the end of the trip.

OK. Now let's switch to the Spaceship reference frame. That is the Earth and the Planet start moving while the Spaceship stays stationary.

First the (moving) Earth starts the trip by moving away from the (stationary) Spaceship. The Earth Twin still sends his signal as soon as the trip begins. The signal to flash the beacon travels at the speed of light.

Now since the Earth Twin is moving, his time appears slower when seen by the stationary Space Twin. And remember that the Earth Twin sent the signal after 4 years of his time.

Now remember the time dilation equation:

t₂ = t₁√1 - (^v / _c)²

In this case, we know the time for the moving Twin, but want to calculate the time the stationary Twin sees. So we must divide the time of the Earth Twin by the Lorentz factor.

t₁ = ^t₂ / _{√1 - (^v / c)²}

And this gives us:

Time for Space Twin = ^{4 years (Earth Twin's Wait)} / _{0.8 (Lorentz Factor)}

= 5 years

So for the Space Twin, the Earth Twin waited 5 years - not 4 - before sending the signal to the beacon. Once sent, the signal travels toward the beacon at the speed of light, 1c.

Also remember that in the Space Twin's reference frame, the distance between the Earth and Twin is not 6.0 light years. And because the Earth and Planet are moving, the distance must be multiplied by the Lorentz factor.

Time for Space Twin = 6 light-years (Earth Twin's Distance) × 0.8 (Lorentz Factor)

= 4.8 light-years

Therefore since the beacon is halfway from the Earth to the Planet, the beacon is

Earth-Beacon Distance (Spaceship Reference): = ^{4.8 light-years} / ₂

= 2.4 light/years

from the Earth and from the Planet.

Now here we have to be very careful with our bookkeeping. We are encountering a situation like we did when considering the Relativity of Simultaneity and have similar calculations to contend with.

Since the beacon is moving toward the approaching radio signal at 0.6 c, and the radio signal is approaching the beacon at 1c, the radio signal reaches the beacon in:

^{2.4 light-years} / _{(1 + 0.6) light-years per year}

= 1.5 years

after the signal is sent from the Earth.

Remember, for the Spaceship the time the Earth Twin delayed sending the signal was 5 years, not 4. And the time it takes the radio signal to reach the beacon is 1.5 years.

So the Space Twin sees the beacon flash in:

5 years + 1.5 years = 6.5 years

after the start of the trip.

But once the beacon flashes, the light beam also travels 2.4 light years toward the Planet. But again be careful! The Planet is also moving toward the light beam at 0.6c. So for the Space Twin the time for the light to reach the Planet is also:

^{2.4 light-years} / _{(1 + 0.6) light-years per year}

= ^{2.4 light-years} / _{1.6 light-years per year}

= 1.5 years

So counting from the start of the trip, the light reaches the Planet in

6.5 years (Time of Flash After Start of Trip) + 1.5 years = 8.0 years.

And as the Planet is moving toward the Spaceship at 0.6c and travels for 4.8 light-years. So the Planet also reaches the Spaceship in:

^{4.8 light-years} / _{0.6 light-years per year}

= 8.0 years

So again we are correct. If the beacon flashes after 5 years as measured by the stationary Space Twin, the Planet reaches the Spaceship at the same time the light reaches the Planet. So the first leg of the trip for by the Space Twin's clock is 8 years.

Exactly as we first saw with the stationary Earth-Planet reference frame.

OK. Here's where things change.

Just what does the Space Twin see is happening for the Earth Twin? Specifically, when does the Space Twin see the light reach the Earth and so mark the end of the first leg - the halfway point - of the whole trip for the Earth Twin?

Well, the Earth is moving away from the light beam at 0.6 c. And the light is catching up at 1c. And since the Earth is 2.4 light-years away at the flash, it takes:

6.5 years (Time of Flash) + ^{2.4 light-years} / _{(1 - 0.6) light-years per year}

= 6.5 years + ^{2.4 light-years}/_{0.4 light-years per years}

= 6.5 years + 6.0 years

= 12.5 years.

Ha????!!!!!!?????? (again Shakespeare). 12.5 years????!!!!!!??????

Yes. 12.5 years.

But we just calculated the first leg for the Space Twin was 8 years!

That is correct.

So you're saying the Planet ends its first half of the trip before the Earth does.

Exactly.

So things are more complicated than ever!

Weeeeellllllllll, not quite.

In fact, things are actually working out nicely.

First we shouldn't be too upset that the Earth and Planet end the first leg of their trips at different times in the Spaceship reference frame. We showed earlier that events which are simultaneous in one reference frame are not simultaneous in another. If the Earth and Planet end the first half of the trip simultaneously in the Earth-Planet reference frame, that need not be true in the Spaceship reference frame.

And besides this extra 4.5 years is just what we need!

For the Spaceship Twin, the Earth Twin doesn't end its first half of the trip for 12.5 years. But we also know that as measured on the Spaceship, time slows down on Earth since in our current reference frame the Earth and Planet are moving and the Spaceship is stationary.

And we know that the amount that time slows down on a moving Earth is calculated by the Lorentz factor. For an Earth traveling at 0.6c the Lorentz factor is 0.8. So the 12.5 year trip as seen by the Space Twin, will mean the Earth-Twin's clock reads:

Trip Duration (for Earth Twin) = 12.5 years (Rocket Reference Frame) × 0.8 (Lorentz factor)

= 10 years

What was that number again?

10 years!

You mean the same time it takes as measured by the Earth Twin if the Earth and Planet are standing still and the Spaceship is moving?

Yep, that's exactly what we mean.

And again for a visualization of our concepts:

Trip Timed by the Beacon: From the Spaceship

(Note: For simplicity we simply show the Planet stopping after the first leg. Actually it would begin its return trip when it reached the spaceship and then return to its starting place. But unless we were wanting to keep track of someone on the planet we do not need to include the Planet specifically in our calculations.)

So with a stationary Spaceship reference frame, we see that everything is consistent with the stationary Earth-Planet reference. Time did indeed slow down on the Moving Earth-Planet system and distances contracted - and by the amount dictated by Special Relativity!

But because of the Relativity of Simultaneity, the Earth Twin keeps going another 4.5 years and so still experiences a 10 year first half of the trip.

That means that both Twins agree that the Earth Twin's clock reads 10 years when the Earth Twin begins his return trip. And they agree that the Space Twin's clock reads 8 years!

Calculating the time for the return trip is more complex in the Spaceship reference frame because of the Relativity of Simultaneity. Because the first leg of the trip does not end at the same time for the Spaceship and Planet, we deviate from the constraints of uniform velocity in a straight line needed for the calculations. The best way to handle it would be simply to have everyone pause after the first leg of the trip for a certain interval. That way the Spaceship, Planet, and Earth "reset" to the same reference frame before beginning the return trip. The second trip would then be a mirror image of the first leg. So the elapsed time during the trip would then be 20 years for the Earth-Planet Reference frame and 16 for the Spaceship.

There's a message to remember as it is central to understanding Relativity. If light acted like baseballs, then light would pick up or lose the velocity of its reference frame. In that case there would be no difference in the times calculated from either a stationary Earth-Planet system or a stationary Spaceship reference. But the speed of light does not pick up or lose any extra velocity from its reference frame, and we know this because it is an experimental fact. The rest is all middle school algebra.

And some people may worry how to interpret what happens when the Planet ends its first leg and starts the return trip and yet the Earth keeps going. It's almost like the space between the Earth and Planet get stretched like a rubber band. But this concept should not be any more of a problem than the idea of the Lorentz contraction - it just goes the other way. Relativity does introduce that strange notion of distortion of space which becomes a key factor in General Relativity and the effect of gravity. But as everything is distorted in proportion, nothing changes within the reference frame itself.

In any case, regardless of which reference frame you choose, the Space Twin is 4 years younger than the Earth Twin at the end of the round trip.

How about that?

 

Et In Conclusione?

As we said at the beginning, if you accept 1) the measured constancy of the speed of light and 2) middle school algebra, then Relativity is an inescapable consequence.

In fact, the high quality of the experimental data and the simplicity of the mathematics is why Relativity was accepted so quickly. By 1919, virtually all physicists accepted what was then still called a "theory", and despite what you may read on - shall we say "certain websites" on the Fount of All Knowledge - they still do.

Every now and then you may hear that someone ran an experiment and found that the difference observed in the speed of light does depend on where you shine the beam. These experiments have inevitably been shown to be non-reproducible by others or that the results don't disprove Relativity after all. And sometimes it's simply that the experimental error has been underestimated, something that's easy to do.

In 1915, Albert published another paper. This was about the General Theory of Relativity. In this case Albert pointed out there was no difference in the force you feel if you accelerate and the force you feel due to gravity. Then using some more math - a bit more advanced than middle school - Albert made some more predictions. One was that we should see light bend if it passes by a massive object.

In 1919, astronomers took some pictures of the stars that show up near the Sun during a total eclipse. The stars weren't exactly where they should have been. But the apparent shift in position was what Albert's theory predicted if light was being bent.

It was also known that the planet Mercury didn't quite behave like Isaac Newton said it should. When it completed an orbit it didn't quite return to where it started. Sure enough, General Relativity predicted the difference, and it was what Albert said it should be. These experiments made headlines and suddenly Albert and the Theory of Relativity was famous.

People kept finding support for Albert's ideas. In 1964, two scientists took a cesium clock - the most accurate kind we have - and put it in a plane. They flew around the Earth and compared the time to a clock that stayed on the ground. Although the changes were in nanoseconds, the differences were there and agreed with what both the Special and General Theories of Relativity.

And more recently the world has found not just support for Albert's theory, but there's actually a practical use for it. For a Global Positioning Satellite to remain at one spot above the surface of the Earth, it actually has to move 3285 miles per hour faster than the rotation of the Earth's surface. And because the satellites are 12,000 miles above the Earth, they experience a different pull of gravity and have to be corrected for both the Special and General Theory of Relativity. So without Albert, the annoying voice would be telling you to "Turn left! Turn left! Turn left!" about five miles from where you're supposed to. And it would get worse each day.

But suppose - just suppose - that someone eventually does show there really is a difference in the speed of light depending on reference frame? Well, if they ever do, then the difference will be so small - tiny, even eentsy - that the basic conclusions of Relativity will still hold. Time will still slow down for a faster moving clock, and length will still get shorter, and simultaneity will still be relative - but just be a smidgen less than Hendrik's equations predict.

In fact, Relativity has hit the point of many correct theories. Eventually they become fact from an epistemological standpoint. So that's why we can legitimately say the Principle of Relativity rather than the Theory of Relativity. After all it's kind of ridiculous to say that Relativity isn't true when the world - including our cars - acts like it is.

But if Relativity still seems a bit too complex and strange - or if all this middle school algebra is a bit too much - as an American president once said:

"Trust me!"

 

References and Further Reading

Einstein: A Life, Denis Brian, Wiley, 1997.

"Einstein Explains Relativity", Life Magazine, April 11, 1938, p. 49.

"Cardinal Sees Atheism's Ghost Behind Relativity", the Miami Herald, April 9, 1929. A complaint that Albert's theory may be promoting atheism - from an American.

The Modern Intellectual Tradition: From Descartes to Derrida, Professor Lawrence Cahoone, Ph.D., Professor of Philosophy, College of the Holy Cross, The Teaching Company. Gives a definition of Relativism which has nothing to do with Relativity.

The Master of Light: A Biography of Albert A. Michelson, Dorothy Michelson Livingston, University of Chicago Press, Chicago (1979). Albert found light didn't change speed depending on the reference frame.

"Ole Roemer and the Speed of Light", American Museum of Natural History, http://www.amnh.org/education/resources/rfl/web/essaybooks/cosmic/p_roemer.html. The first measurement of the speed of light.

"Einstein at the Patent Office", Swiss Federal Institute of Intellectual Property, https://www.ige.ch/en/about-us/institute.html. Nice bio of Albert's early years.

How Does Relativity Theory Resolve the Twin Paradox?, Ronald Lasky, Scientific American, March 17, 2003. This article explains the consistency of the observations of the Earth and Space Twins. It also shows you - again in an interesting manner - what the Twins would actually see in real time.

However, as one commenter posted, there was no explanation of the actual question always asked - how to reconcile the Twin Paradox if the Spaceship is treated as stationary. We have seen in our own essay that you must introduce the Relativity of Simultaneity - and, alas, the extra explanation lengthens the size of the essay beyond what is desired for modern magazine articles.

Viva la Internet and online publishing!

Relativity: An Introduction to Spacetime Physics, Steve Adams, Taylor and Francis, 1997. A comprehensive introduction of both the Special and General Theory.

"Scientist and Mob Idol", Alva Johnston, The New Yorker, December 2 - 7, 1933. A rather rambling and not terribly informative "profile" of Albert Einstein. But we see that claims of Albert being famous only after World War II are not correct.

 

Return to Merry History Table of Contents

Return to CooperToons Home Page